How Much Insulation Do One Necessitate For A X×16 Shed?
The total of insulation that you require for x×sixteen shed depends on things like the roof blueprint, stud size, too stud spacings. Because of this, the amount can vary.
The following provides you with a detailed footstep-past-stride breakdown of how to calculate the sum of insulation needed for the walls as well as roof of the shed.
However, if you lot are not interested in the calculations, then skip to the final section. There yous will notice tables for the sum of insulation needed for 10×xvi sheds alongside specific roof designs, stud sizes, as well as stud spacings.

To insulate a ten×xvi shed, some 477.01 ft2 to 532.37 ft2 of insulation is needed, depending on the stud size, stud spacing, and roof design.
Calculating Actual Wall Area Requiring Insulation
Ceiling Height Must Be Considered
You tin can mensurate your exact ceiling meridian as well as brand adjustments to the calculations presented below, just nosotros are going to function based on the fact that the shed has a measure ceiling meridian of 8 ft.
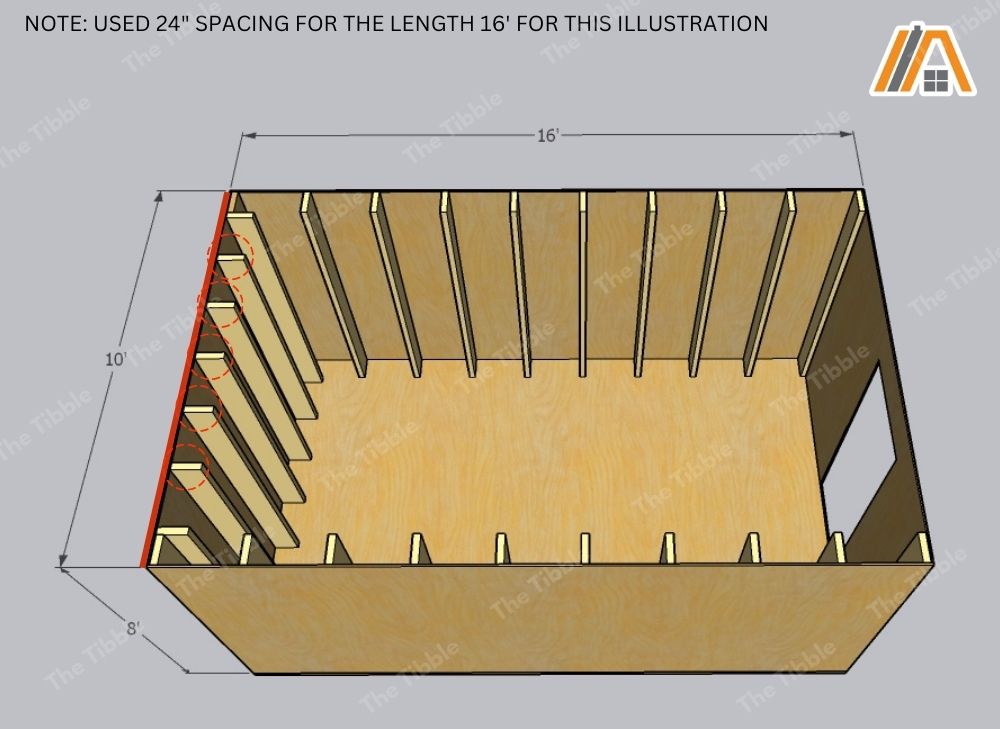
Understanding the ten×16 Measurements
The 10×16 measurements are the outside measurements of the shed. The inside measurements ask to live adjusted for the presence of stud function.

We will be calculating the internal measurements. We volition not be accounting for doors as well as windows. The areas of these are slow to calculate, then y'all can add together upward the total area taken up past these, depending on the number as well as place in addition to subtract this from the total surface area.
Stud Sizes
Along each 16-ft wall, y'all are going to lose 2 in. at each end for the kickoff together with concluding studs. This cuts the insulation surface area from 128 ftii (16 ft ten viii ft) to 125.33 ftii according to the following:
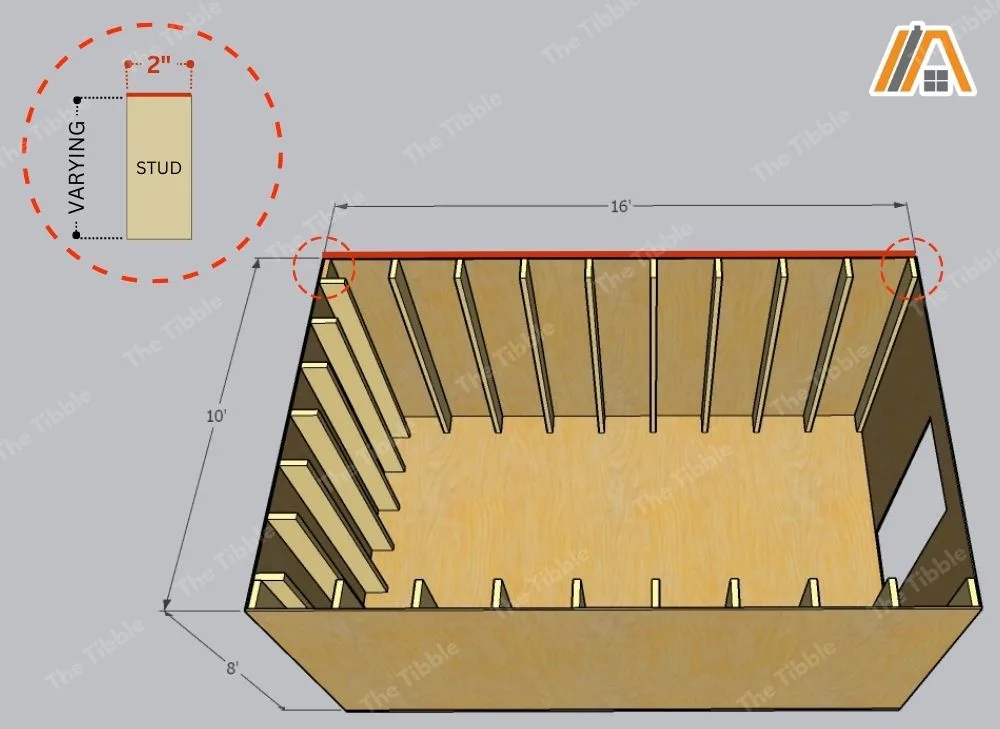
Calculation (1a): 16 ft ten 12 = 192 inward.
Calculation (1b): 192 in. – (two + ii) in = 188 inward.
Calculation (1c): 188 inward. /12 = 15.67 ft
Calculation (1d): 15.67 ft ten viii ft = 125.33 fttwo
Along each x-ft wall, y'all are too going to lose two in. at each terminate for the outset too terminal studs. This cuts the insulation area from fourscore ft2 (ten ft ten viii ft) to 77.33 ftii according to the next:
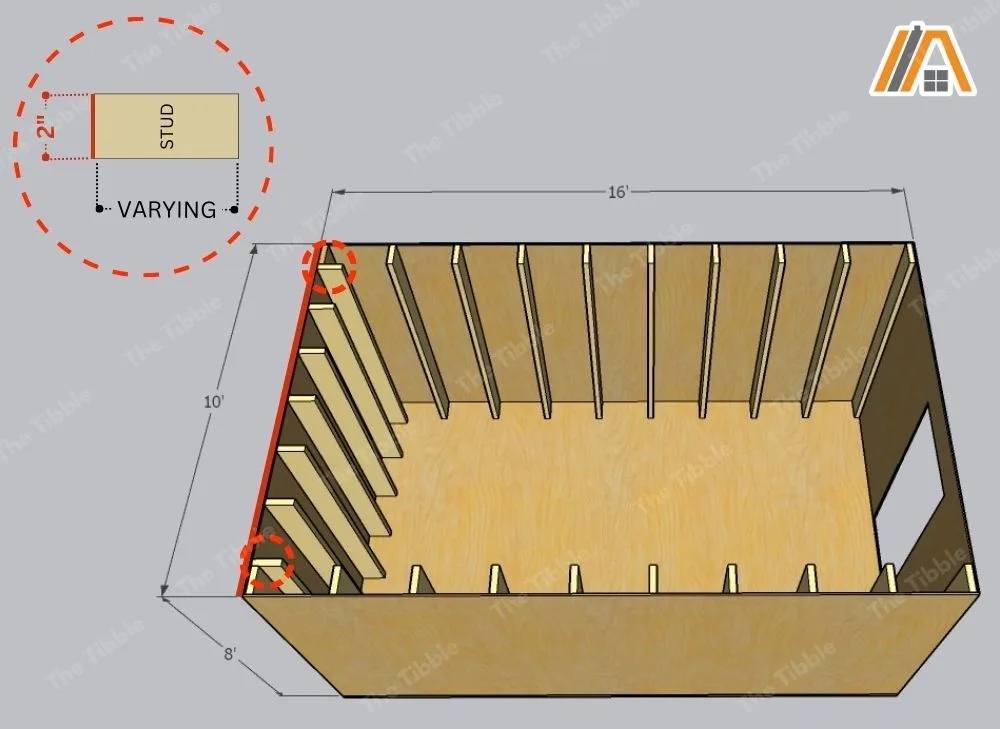
Calculation (2a): 10 ft ten 12 = 120 inwards.
Calculation (2b): 120 inwards. – (two + two) inwards = 116 inward.
Calculation (2c): 116 inward. /12 = nine.67 ft
Calculation (second): 9.67 ft 10 viii ft = 77.33 ft2
In improver, yous volition lose the depth of i stud at each stop. This accounts for the cease studs of the long walls. The amount of space lost hither depends on the size of the studs you lot function.
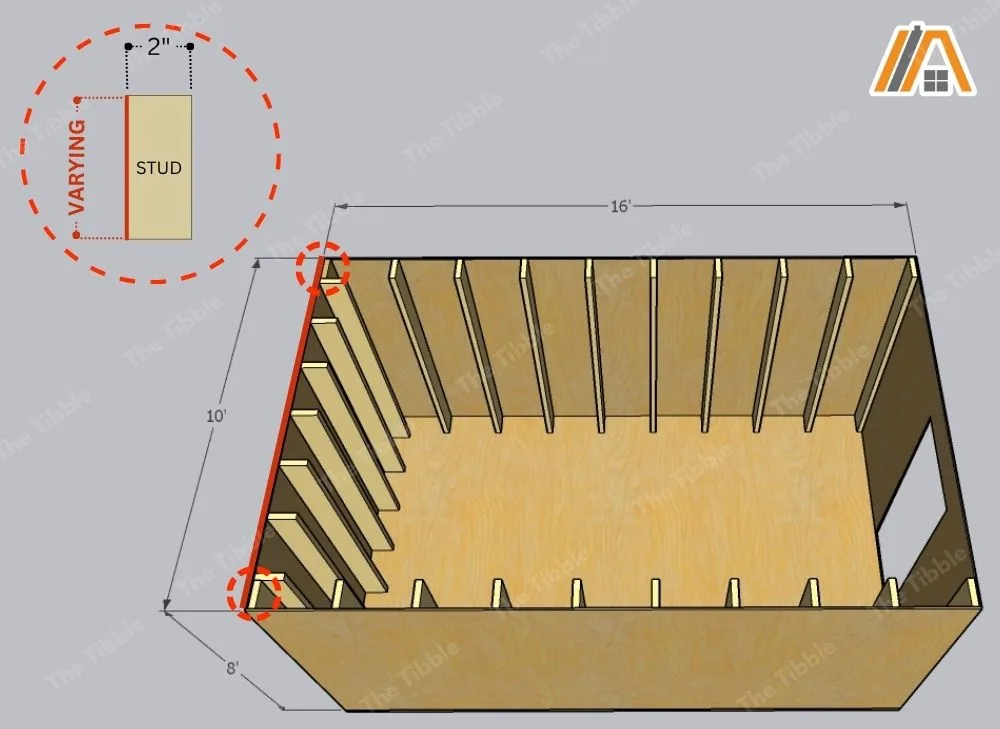
I imagine that for a shed, you lot’ve probably used ii×iv, ii×six, or ii×viii studs. Anything larger too yous lose also much infinite, specially considering that in that location is no minimum insulation thickness/R-value requirements for a shed.
ii×4, two×vi, or two×eight studs agency a loss of 8 inwards., 12 inwards., or sixteen in., respectively.
Let’second plug these figures inwards the to a higher place equations. We will deduct them from the length inward inches (given inwards Calculation (2b)).
For each x-pes wall:
| New length | New length inwards feet | New area | |
| ii×four studs | 116 in. – eight inward. = 108 inward. | 180 inward. /12 = 9 ft | 9 ft ten eight ft = 72 ft2 |
| ii×vi studs | 116 inwards. – 12 inwards. = 104 inward. | 104 inwards. /12 = 8.67 ft | viii.67 ft ten viii ft = 69.33 ft2 |
| two×8 studs | 116 inwards. – xvi in. = 100 in. | 100 in. /12 = viii.33 ft | viii.33 ft x eight ft = 66.67 ft2 |
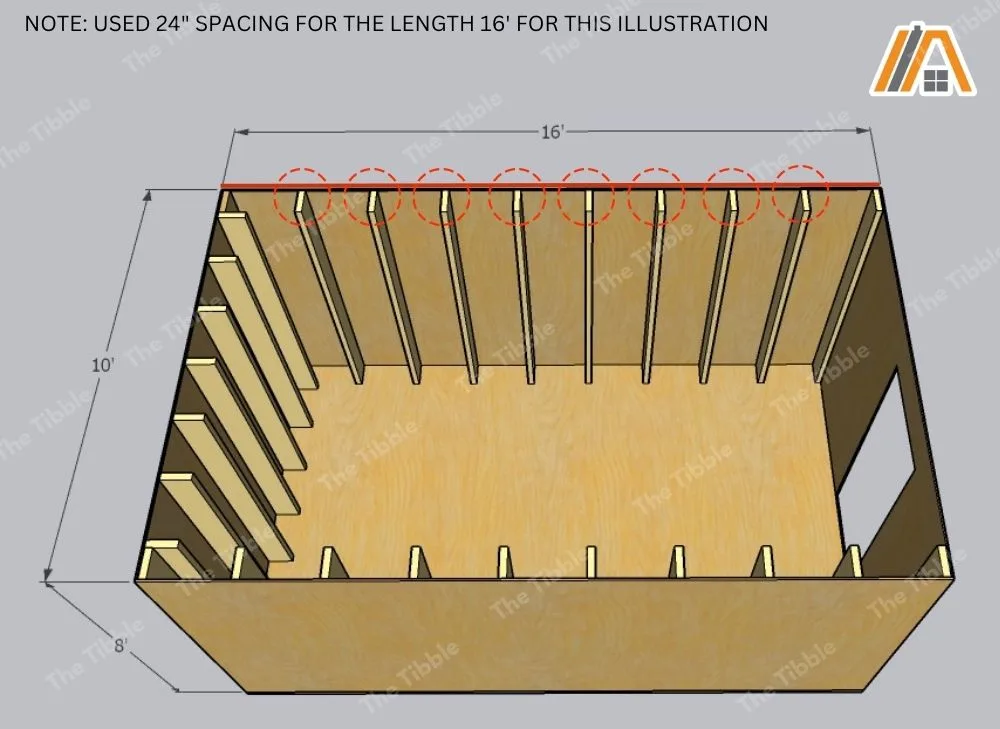
Stud Spacing
Then, of grade, you must deduct the area used up by the residue of the studs along the wall. This depends on the number of studs used, together with the number of studs used depends on the stud spacing chosen.
The recommended stud spacing for a shed wall is sixteen-24 in.
We’re going to expect at the length available between the two stop studs. So, for each 16-ft wall, this is 188 in. (Calculations (1b)) and for each 10-ft wall, it is 108 in., 104 inwards., in addition to 100 inward., depending on the stud size (Table i).
For each 16-ft wall:
| sixteen inwards. stud spacing | xviii inwards. stud spacing | 20 inwards. stud spacing | 22 in. stud spacing | 24 in. stud spacing |
| 11.75 studs | x.44 studs | nine.four studs | viii.55 studs | 7.83 studs |
| In existent life, you would role xi studs | In existent life, y'all would role 10 studs | In real life, yous would use ix studs | In existent life, you would use ix studs | In existent life, you lot would purpose 8 studs |
For each ten ft wall:
| xvi in. stud spacing | 18 inward. stud spacing | twenty inward. stud spacing | 22 inward. stud spacing | 24 in. stud spacing | |
| two×four studs | vi.75 studs (inwards existent life, you lot would use half-dozen studs) | half-dozen studs | 5.four studs (inward real life, yous would use v studs) | iv.ix studs (in existent life, yous would purpose v studs) | four.v studs (inward existent life, you lot would purpose five studs) |
| two×vi studs | half dozen.v studs (inwards existent life, you would purpose half dozen studs) | five.78 studs (in real life, you lot would use 5 studs) | v.ii studs (inward real life, you lot would use five studs) | iv.73 studs (in real life, yous would role five studs) | iv.33 studs (inward real life, y'all would role v studs) |
| 2×8 studs | 6.25 studs (inwards real life, y'all would purpose vi studs) | five.56 studs (inward real life, yous would role v studs) | 5 studs | iv.55 studs (inward existent life, you would role five studs) | iv.17 studs (inward real life, you would use five studs) |
Total Wall Area to Cover
For each additional stud, you are going to subtract ii in. from the total wall width. Multiplying this past the top volition give you lot the full wall expanse needed to be covered by insulation.
For the 16-ft walls:
| Width excluding studs | Area requiring insulation (per wall) | Area requiring insulation (both walls) | |
| eleven studs | 166 inward. = thirteen.83 ft | 110.67 ft2 | 221.34 fttwo |
| x studs | 168 inwards. = fourteen ft | 112 fttwo | 224 ft2 |
| ix studs | 170 in. = xiv.17 ft | 113.33 ft2 | 226.66 ftii |
| viii studs | 172 in. = 14.33 ft | 114.67 fttwo | 229.34 fttwo |
For the ten-ft walls:
| Width excluding studs | Area requiring insulation (per wall) | Area requiring insulation (both walls) | |
| half-dozen studs | two×four: 96 inward. = eight ft ii×six: 92 inward. = 7.67 ft two×eight: 88 inwards. = 7.33 ft | ii×four: 64 ftii 2×6: 61.33 ft2 2×viii: 58.67 ft2 | two×4: 128 fttwo ii×six: 122.66 ftii ii×viii: 117.34 ftii |
| 5 studs | 2×four: 98 inward. = viii.17 ft two×6: 94 inwards. = vii.83 ft ii×eight: ninety inward. = seven.five ft | two×iv: 65.33 ft2 2×half-dozen: 62.67 ftii 2×8: lx fttwo | two×iv: 130.66 ft2 2×vi: 125.34 ft2 two×8: 120 ft2 |
For the walls inward full:
| Stud spacing | two×4 studs | 2×6 studs | ii×8 studs |
| 16 inwards. | 221.34 ft2 + 128 fttwo = 349.34 ft2 | 221.34 fttwo + 122.66 ft2 = 344 fttwo | 221.34 fttwo + 117.34 fttwo = 338.68 ftii |
| xviii inward. | 224 fttwo + 128 ft2 = 352 ft2 | 224 ft2 + 125.34 ft2 = 349.34 ftii | 224 ftii + 120 ft2 = 344 ft2 |
| twenty inwards. | 226.66 ft2 + 130.66 ft2 = 357.32 fttwo | 226.66 ftii + 125.34 ftii = 352 fttwo | 226.66 fttwo + 120 ftii = 346.66 fttwo |
| 22 in. | 226.66 ftii + 130.66 ftii = 357.32 ftii | 226.66 fttwo + 125.34 ft2 = 352 ft2 | 226.66 ftii + 120 fttwo = 346.66 ft2 |
| 24 inward. | 229.34 ftii + 130.66 ft2 = 360 ftii | 229.34 ftii + 125.34 ftii = 354.68 ft2 | 229.34 fttwo + 120 ftii = 349.34 ft2 |
Calculating Actual Roof Area Requiring Insulation
For the roof, y'all besides take to calculate the area minus the rafters.
However, things tin can get a fiddling complicated because yous take to accept into business relationship the roof blueprint (gable, skillion, or flat). If yous accept a skillion or gable roof, the pitch adds additional expanse.
Flat Roofs
The surface area of the apartment roof without rafters is going to live:
Calculation (3a): 10 ft ten sixteen ft = 160 fttwo
However, y'all will live installing rafters, and so nosotros involve to deduct the widths of these—insulation is exclusively installed betwixt the rafters.
Each rafter is two inwards. wide, installed parallel to the short border (if you lot stood at the brusk end, you lot would encounter them crossing left to right), too the recommended rafter spacing for sheds is 16 inward.
This way that we are looking at a starting width of:
Calculation (3b): sixteen ft x 12 = 192 inwards.
Let’s deduct the width of the ii cease rafters every bit these have to live at the end as well as the residue of the rafters volition be spaced betwixt them.
Calculation (3c): 192 in. – four in. = 188 inwards.
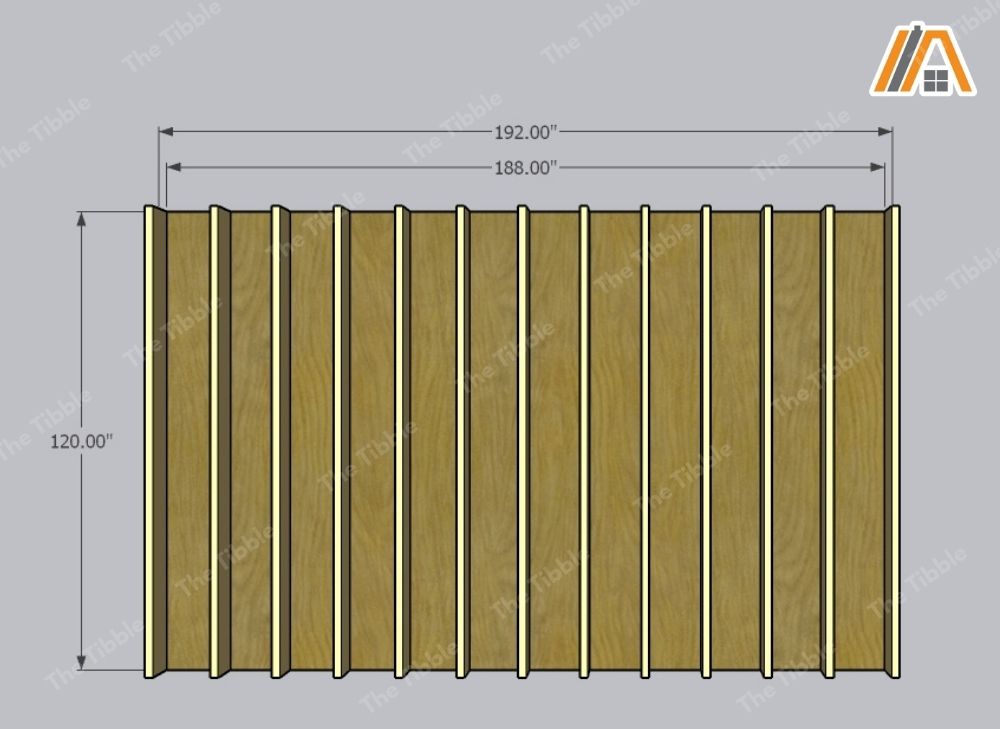
With a recommended spacing of 16 in., yous volition necessitate 11.75 rafters (Table two). Now, you can’t accept a fraction of a rafter, so we’re going to round this downwardly to xi rafters.
You tin circular upwards to 12 if you lot choose, peculiarly if you lot are opting for a heavier roofing fabric, only eleven rafters should live sufficient in almost cases.
Eleven rafters betwixt the ii terminate ones means an additional loss of:
Calculation (3d): 11 ten ii in. = 22 in.
Using this, nosotros can calculate the expanse that insulation volition live required to comprehend a flat roof for a 10×sixteen shed:
Calculation (4a): 188 inward. – 22 in. = 166 inward.
Calculation (4b): 166 in. /12 = xiii.83 ft
Calculation (4c): 13.83 ft x ten ft = 138.33 fttwo
Gable Roofs
Roof Panels
The start thing that we have to look at here is the pitch. The minimum pitch you need is a 1/iv inwards. increment inwards rising (the distance from the ceiling business to the roof) for every human foot of function (the distance from the edge of the edifice to the eye of it).
However, this pitch way an angle of entirely 1.nineteen°, which is unlikely to be the pitch y'all choose for your gable-roofed shed.
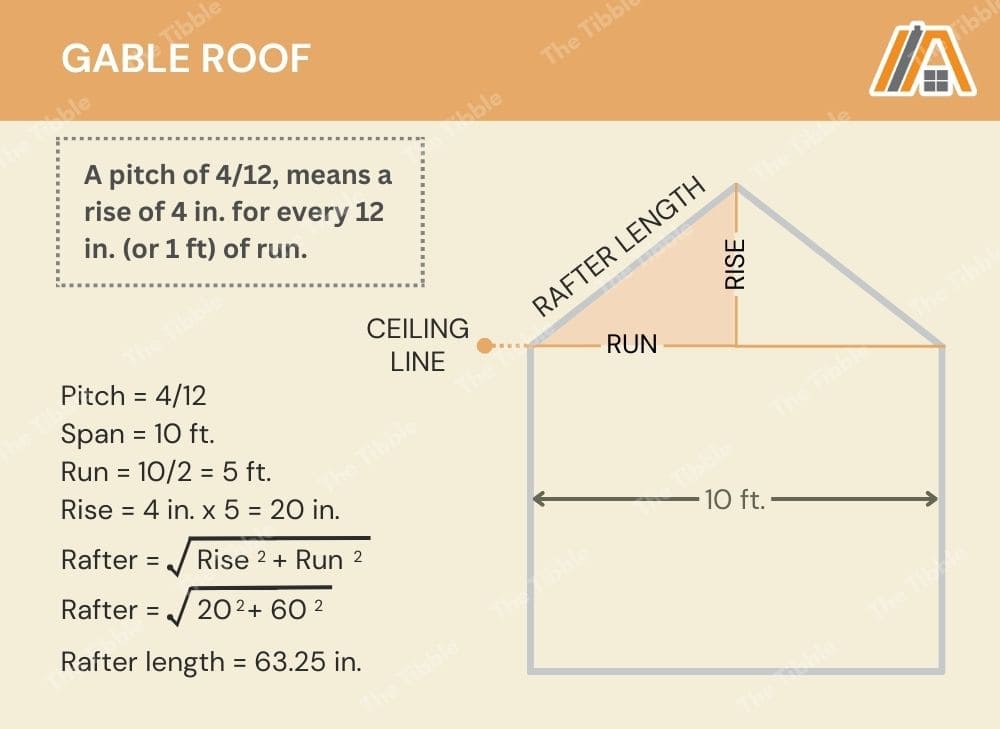
Instead, nosotros volition wait at a pitch of four/12, which means a rise of four in. for every 12 inwards. (or 1 ft) of operate. This seems to live a common pitch used for gable-roofed sheds.
For a ten×16 shed, the function is:
Calculation (5a): x ft /two = five ft
So, there are v 10 ane ft lengths, which way the rising is calculated to be:
Calculation (5b): 4 inwards. 10 5 = 20 inwards.
Using pythagoras’ theorem, nosotros tin can use this to calculate the rafter length (represented every bit the hypotenuse).
Calculation (6a): Hypotenuse = √(next)ii + (reverse)ii
Calculation (6b): C = √(A)2 + (B)two
Calculation (6c): C = √(xx)ii + (60)2
Calculation (6d): C = √400 + 3600
Calculation (6e): C = √4000
Calculation (6f): C = 63.25 in.
The rafter length upward each side of the roof is, hence, 63.25 inward. or 5.27 ft.
The rafter spacing that is used for apartment roofs is besides used for gabled roofs. So, each side has two + 11 rafters, each alongside a width of 2 in.
We have already done this calculation in the section on flat roofs (Calculation (4b)). The roof width less the width of the rafters is xiii.83 ft.
Thus, the area of each side that needs to live covered in insulation is:
Calculation (7a): 5.27 ft 10 13.83 ft = 72.88 ft2
And the total area of the roof panels that required insulation roofing is:
Calculation (7b): 72.88 ft2 10 2 = 145.76 ft2
Gable Spaces
As the roof has a tiptop, there are ii triangles of space betwixt the short walls in addition to the apex of the roof. These spaces as well needs to be insulated.
The space is reduced by the broad side of the rafters on each side of the meridian, the wide side of a rafter set up along the top of the wall (equally if y'all were laying the starting time joist for a ceiling), as well as at to the lowest degree one upright beam that is set up alongside the broad side out as well as which bisects the triangle.

Because all of these beams are facing broad side out, the size of the beams comes into play.
You volition most probable role 2×iv or 2×half-dozen rafters.
The ascension of xx inward. (Calculation (5b)) is reduced past:
- viii in. at the height as well as bottom for 2×four rafters
- 12 inward. at the summit and bottom for two×6 rafters
This way that the rise of the infinite is:
- 12 in. if you lot are using 2×iv rafters
- viii in. if you lot are using two×6 rafters
While the rafters trim down the internal area of the triangular gable infinite, because they are all either ii×iv or two×vi, it does not change the angles. (Triangles are bully like that).
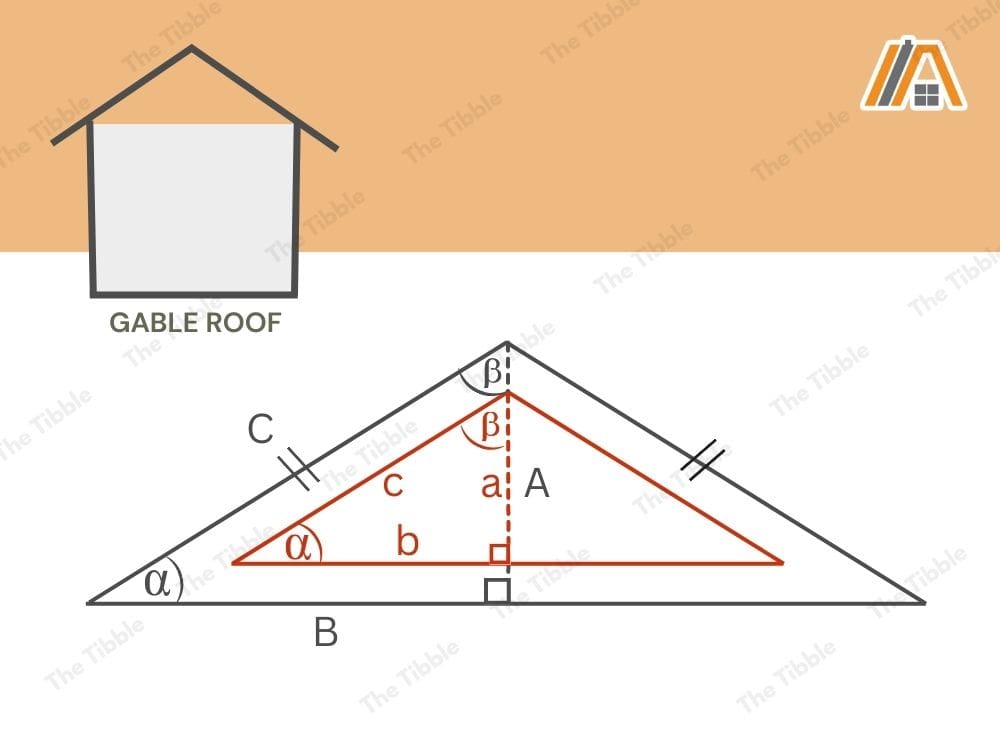
To calculate the surface area of these gable triangles, we involve to know the values of a and b inward the to a higher place diagram.
Here’s what we know:
- A = xx inward. (Calculation (5b))
- B = five ft = 60 inwards. (Calculation (5a))
- C = 63.25 inwards. (Calculation (6f))
We tin calculate α using trigonometry:
Calculation (8a): sin(α) = opposite/hypotenuse
Calculation (8b): sin(α) = A/C
Calculation (8c): sin(α) = twenty/63.25
Calculation (8d): sin(α) = 0.316205533
Calculation (8e): α = sin-one(0.316205533)
Calculation (8f): α = eighteen.43°
As stated previously, the angles of the larger triangle are the same for the smaller i.
To calculate the length of a, nosotros involve to subtract the height in addition to bottom rafter dimensions.
I want to bank note here that this is not a fully accurate measuring because the heart parallel beam way that nosotros are non really mensuration through the proper central signal.
However, one time we have completed the residual of the calculations, I notice it makes minimal divergence.
So, for two×4 rafters:
Calculation (9a): a = A – (ii 10 four in.)
Calculation (9b): a = twenty in. – 8 in.
Calculation (9c): a = 12 inwards.
And for two×6 rafters:
Calculation (10a): a = A – (ii ten six in.)
Calculation (10b): a = 20 inward. – 12 in.
Calculation (10c): a = eight in.
The area of a triangle is calculated as (base of operations x height)/two. Base is b together with elevation is a. We know a, then nosotros postulate to calculate b. We are going to make this using more than trigonometry.
For two×four rafters:
Calculation (11a): tan (α) = reverse/adjacent
Calculation (11b): tan (α) = a/b
Calculation (11c): tan (α) = 12/b
Calculation (11d): tan (xviii.43) = 12/b
Calculation (11e): 0.333237365 = 12/b
Calculation (11f): b = 12/0.333237365
Calculation (11g): b = 36.01 in.
From b, nosotros are going to subtract one-half the parallel beam:
Calculation (11h): b = 36.01 inward. – two inwards.
Calculation (11i): b = 34.01 inward.
For 2×half-dozen rafters:
Calculation (12a): tan (α) = contrary/next
Calculation (12b): tan (α) = a/b
Calculation (12c): tan (α) = eight/b
Calculation (12d): tan (xviii.43) = 8/b
Calculation (12e): 0.333237365 = eight/b
Calculation (12f): b = eight/0.333237365
Calculation (12g): b = 24.01 inwards.
Again, nosotros accommodate for the fundamental beam:
Calculation (12h): b = 24.01 in. – three inward.
Calculation (12i): b = 21.01 in.
Now we tin calculate the surface area of the triangles.
Remember that this is alone for one side of the primal parallel beam, and then nosotros take to double it at the cease. Since the concluding stride is to dissever by 2, we can essentially skip this stride, merely I volition include it anyway.
Then, we have to double the issue once more for the contrary gable.
| ii×4 rafters | ii×vi rafters | |
| Area of half the gable | (base of operations x superlative) /two | (base of operations x meridian) /ii |
| (b 10 a) /ii | (b 10 a) /2 | |
| (34.01 inwards. x 12 inwards.) /two | (21.01 in. 10 viii inward.) /2 | |
| 408.12 in.two /2 | 168.08 inwards.two /ii | |
| 204.06 in.2 | 84.04 in.2 | |
| Area of one gable | 204.06 inwards.two x 2 | 84.04 in.ii ten 2 |
| 408.12 in.two | 168.08 inwards.two | |
| Area of i gable in fttwo | 2.83 fttwo | one.17 ft2 |
| Total gable area | ii.83 ft2 ten 2 | i.17 fttwo 10 ii |
| v.66 ft2 | 2.34 fttwo |
Total Gable Roof Area Requiring Insulation
| 2×iv rafters | 2×half-dozen rafters | |
| Roof panels | 145.76 fttwo | 145.76 ftii |
| Gable spaces | 5.66 ft2 | 2.34 ft2 |
| Total expanse | 151.42 ftii | 148.ane fttwo |
Skillion Roofs
Roof Panel
Again, we start alongside pitch. Skillion roofs are not going to live equally steeply pitched equally gable roofs. A common pitch angle for these shed roofs is ten°, so this is what we volition function for the calculations.
You can ever insert your actual roof pitch into the equations to calculate the surface area for your roof if it is non ten°.
We showtime ask to calculate the length of the roof. This is the triangle hypotenuse (C) equally illustrated below.
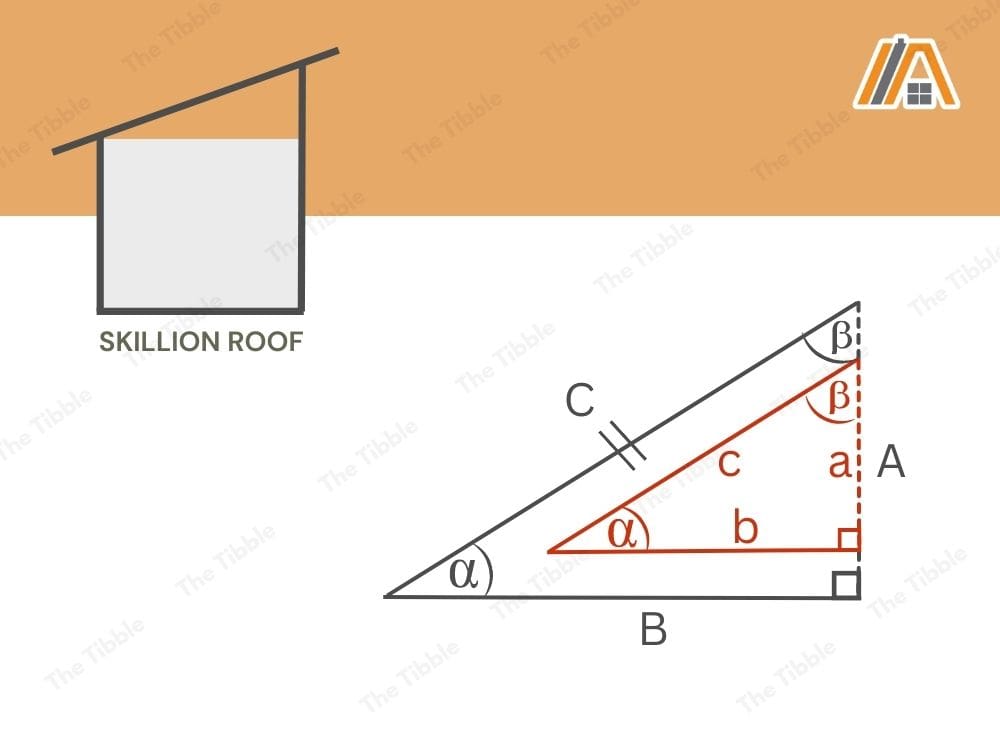
We know the angle α (x°) in addition to the dimension B (ten ft). This means that we tin can role trigonometry to calculate C.
Calculation (13a): cos(α) = adjacent /hypotenuse
Calculation (13b): cos(α) = B /C
Calculation (13c): cos(x) = 10 ft /C
Calculation (13d): 0.984807753 = x ft /C
Calculation (13e): C = x ft /0.984807753
Calculation (13f): C = ten.fifteen ft
Using this in addition to the known width of 16 ft, nosotros tin calculate the area of the roof panel without rafters.
Calculation (14a): ten.xv ft 10 16 ft = 162.4 ftii
But at that place are almost sure enough rafters, and so we call for to suit for these.
Each rafter is ii in. in width. The same spacing applies equally before (sixteen inward.). We take really already calculated the width of the roof minus the the width of the rafters (Calculations (3b), (3c), (3d), (4a), too (4b)).
This width is 13.83 ft. The surface area of skillion roof panels for a x×sixteen shed requiring insulation covering is:
Calculation (14b): ten.fifteen ft 10 13.83 ft = 140.37 ft2
Gable Spaces
Even though this is non a gable roof, there are withal those triangles on the side that ask to live insulated.
We involve to calculate the expanse of the small triangle inwards the sketch in a higher place. The expanse is decreased by the broad side of a rafter beam along the border of the roof panel, the acme of the wall at the ceiling line of work, together with the rising.
Again, nosotros can calculate a every bit A minus the breadth of the beam at the tiptop as well as bottom of the infinite. This means it is time to view the rafter sizes (2×four and ii×6).
This fourth dimension, nosotros don’t actually know A, and then we necessitate to beginning here:
Calculation (15a): tan(α) = contrary /adjacent
Calculation (15b): tan(α) = A /B
Calculation (15c): tan(x) = A /ten ft
Calculation (15d): 0.17632698 = A /10 ft
Calculation (15e): A = 10 ft ten 0.17632698
Calculation (15f): A = i.76 ft
Let’second convert this to inches so that nosotros tin can subtract the rafter breadths (given in inches). 1.76 ft is 21.12 inward.
Calculating a for ii×iv rafters:
Calculation (16a): a = A – (2 ten 4 inward.)
Calculation (16b): a = 21.12 in. – eight inwards.
Calculation (16c): a = xiii.12 in.
Calculation (16d): xiii.12 inwards. /12 = i.09 ft
Calculating a for two×half-dozen rafters:
Calculation (17a): a = A – (ii ten 6 inwards.)
Calculation (17b): a = 21.12 inwards. – 12 inward.
Calculation (17c): a = nine.12 in.
Calculation (17d): ix.12 inwards. /12 = 0.76 ft
To detect the area of the pocket-sized triangle, we as well ask to know the base of operations length, which is b.
For two×4 rafters:
Calculation (18a): tan(α) = reverse /next
Calculation (18b): tan(α) = a /b
Calculation (18c): tan(x) = i.09 ft /b
Calculation (18d): 0.17632698 = one.09 ft /b
Calculation (18e): b = 1.09 ft /0.17632698
Calculation (18f): b = six.eighteen ft
For two×vi rafters:
Calculation (19a): tan(α) = contrary /next
Calculation (19b): tan(α) = a /b
Calculation (19c): tan(ten) = 0.76 ft /b
Calculation (19d): 0.17632698 = 0.76 ft /b
Calculation (19e): b = 0.76 ft /0.17632698
Calculation (19f): b = four.31 ft
Now we tin calculate the expanse of ane gable infinite as well as multiply it past 2 (to account for the contrary infinite) to get the full gable area.
| ii×iv rafters | ii×half-dozen rafters | |
| Area of the gable space | (base of operations ten elevation) /ii | (base of operations ten height) /2 |
| (b x a) /two | (b x a) /two | |
| (half-dozen.xviii ft x 1.09 ft) /two | (4.31 ft 10 0.76 ft) /two | |
| half-dozen.74 ftii /ii | 3.28 ft2 /ii | |
| three.37 fttwo | ane.64 fttwo | |
| Total gable area | 3.37 ft2 ten two | one.64 fttwo x ii |
| half dozen.74 ft2 | iii.28 fttwo |
Wall Extension
We’re not done still! With skillion roofs, we besides accept to insulate the side of the roof that is essentially an extension of the i xvi-ft wall.
Happily, this is a straightforward calculation.
The wall extension is 16 ft broad, less the stud widths. We already have these calculated inwards Table four:
- When eleven studs are used (xvi inwards. stud spacing), the width is xiii.83 ft
- When x studs are used (xviii inwards. stud spacing), the width is 14 ft
- When nine studs are used (twenty inwards. together with 22 inwards. stud spacing), the width is xiv.17 ft
- When eight studs are used (24 in. stud spacing), the width is 14.33 ft
The meridian is equal to the rising (A), which is one.76 ft.
So, the total wall extension expanse requiring insulation coverage is:
| Area | |
| eleven studs; sixteen inward. stud spacing | one.76 ft ten thirteen.83 ft = 24.34 ft2 |
| 10 studs; eighteen inwards. stud spacing | ane.76 ft ten xiv ft = 24.64 ft2 |
| nine studs; twenty inward. together with 22 inwards. stud spacing | i.76 ft ten fourteen.17 ft = 24.94 ftii |
| eight studs; 24 inwards. stud spacing | 1.76 ft 10 14.33 ft = 25.22 ftii |
Total Skillion Roof Area in addition to New Wall Area
For ease, nosotros will comprise the wall extension expanse into the total wall expanse instead of the total roof area. Below is an extension of Table six.
| Stud spacing | 2×four studs | ii×six studs | 2×viii studs |
| xvi in. | 349.34 ft2 + 24.34 ftii = 373.68 ftii | 344 ftii + 24.34 fttwo = 368.34 ft2 | 338.68 ftii + 24.34 fttwo = 363.02 ft2 |
| 18 inwards. | 352 ftii + 24.64 ftii = 376.64 ftii | 349.34 fttwo + 24.64 fttwo = 373.98 ft2 | 344 ftii + 24.64 fttwo = 368.64 ft2 |
| xx in. | 357.32 fttwo + 24.94 ftii = 382.26 fttwo | 352 ft2 + 24.94 ft2 = 376.94 fttwo | 346.66 fttwo + 24.94 fttwo = 371.six ft2 |
| 22 inward. | 357.32 ft2 + 24.94 ft2 = 382.26 ftii | 352 ftii + 24.94 ft2 = 376.94 ftii | 346.66 ft2 + 24.94 fttwo = 371.6 ft2 |
| 24 inward. | 360 fttwo + 25.22 ft2 = 385.22 ft2 | 354.68 ftii + 25.22 ftii = 379.9 ft2 | 349.34 ft2 + 25.22 ftii = 374.56 fttwo |
Now for the full skillion roof expanse for x×xvi shed:
| Roof panel surface area | Gable infinite expanse | Total roof area | |
| 2×4 | 140.37 fttwo | half-dozen.74 fttwo | 147.eleven fttwo |
| 2×half-dozen | 140.37 fttwo | 3.28 ft2 | 143.65 ft2 |
Amount of Insulation Required to Insulate x×sixteen Shed
Flat Roof
2×4 Studs
| Stud spacing | Wall area | Roof surface area | Total expanse |
| 16 inwards. | 349.34 ft2 | 138.33 ft2 | 487.67 ft2 |
| eighteen inwards. | 352 ft2 | 138.33 ftii | 490.33 ftii |
| twenty inwards. | 357.32 ft2 | 138.33 ft2 | 495.65 ftii |
| 22 inwards. | 357.32 fttwo | 138.33 ftii | 495.65 fttwo |
| 24 inwards. | 360 ft2 | 138.33 fttwo | 498.33 ft2 |
two×vi Studs
| Stud spacing | Wall area | Roof area | Total area |
| sixteen inwards. | 344 ftii | 138.33 fttwo | 482.33 fttwo |
| 18 inward. | 349.34 fttwo | 138.33 ft2 | 487.67 ft2 |
| twenty inward. | 352 ftii | 138.33 ft2 | 490.33 fttwo |
| 22 in. | 352 fttwo | 138.33 fttwo | 490.33 fttwo |
| 24 inward. | 354.68 ftii | 138.33 ft2 | 493.01 ftii |
two×viii Studs
| Stud spacing | Wall area | Roof area | Total area |
| sixteen in. | 338.68 fttwo | 138.33 fttwo | 477.01 fttwo |
| 18 inwards. | 344 ft2 | 138.33 ft2 | 482.33 fttwo |
| xx in. | 346.66 ft2 | 138.33 ft2 | 484.99 ft2 |
| 22 inward. | 346.66 ftii | 138.33 ft2 | 484.99 ft2 |
| 24 inwards. | 349.34 ft2 | 138.33 fttwo | 487.67 fttwo |
Gable Roof
2×4 Rafters together with 2×iv Studs
| Stud spacing | Wall surface area | Roof expanse | Total expanse |
| xvi inward. | 349.34 ft2 | 151.42 fttwo | 500.76 fttwo |
| xviii inward. | 352 fttwo | 151.42 ft2 | 503.42 ft2 |
| xx in. | 357.32 fttwo | 151.42 ftii | 508.74 ft2 |
| 22 in. | 357.32 fttwo | 151.42 fttwo | 508.74 fttwo |
| 24 in. | 360 ft2 | 151.42 ft2 | 511.42 ftii |
ii×iv Rafters together with ii×half-dozen Studs
| Stud spacing | Wall surface area | Roof expanse | Total expanse |
| 16 inwards. | 344 ft2 | 151.42 ft2 | 495.42 fttwo |
| eighteen inward. | 349.34 fttwo | 151.42 ftii | 500.76 ft2 |
| xx inward. | 352 ft2 | 151.42 fttwo | 503.42 ftii |
| 22 inwards. | 352 ftii | 151.42 ft2 | 503.42 fttwo |
| 24 inwards. | 354.68 ft2 | 151.42 ft2 | 506.i fttwo |
two×four Rafters in addition to two×eight Studs
| Stud spacing | Wall area | Roof area | Total surface area |
| sixteen in. | 338.68 ftii | 151.42 ftii | 490.01 ft2 |
| eighteen in. | 344 fttwo | 151.42 ft2 | 495.42 fttwo |
| twenty in. | 346.66 ft2 | 151.42 fttwo | 498.08 ftii |
| 22 inward. | 346.66 fttwo | 151.42 fttwo | 498.08 ftii |
| 24 in. | 349.34 ft2 | 151.42 fttwo | 500.76 ftii |
ii×six Rafters in addition to ii×4 Studs
| Stud spacing | Wall expanse | Roof area | Total surface area |
| sixteen in. | 349.34 ftii | 148.one fttwo | 497.44 ftii |
| eighteen inward. | 352 ft2 | 148.one fttwo | 500.1 fttwo |
| 20 inward. | 357.32 ftii | 148.i ftii | 505.52 fttwo |
| 22 inward. | 357.32 fttwo | 148.ane fttwo | 505.52 ftii |
| 24 inwards. | 360 ft2 | 148.one fttwo | 508.i ft2 |
two×six Rafters as well as ii×6 Studs
| Stud spacing | Wall expanse | Roof expanse | Total expanse |
| sixteen in. | 344 ftii | 148.ane ft2 | 492.one ft2 |
| eighteen in. | 349.34 ft2 | 148.i fttwo | 497.44 ftii |
| 20 in. | 352 ft2 | 148.1 ftii | 500.i ftii |
| 22 inward. | 352 ftii | 148.ane ft2 | 500.one ft2 |
| 24 in. | 354.68 ft2 | 148.i ftii | 502.48 ft2 |
ii×6 Rafters too ii×8 Studs
| Stud spacing | Wall area | Roof surface area | Total surface area |
| xvi inwards. | 338.68 fttwo | 148.ane ftii | 486.78 fttwo |
| xviii inward. | 344 ftii | 148.ane ft2 | 492.1 ftii |
| xx inwards. | 346.66 ft2 | 148.one ft2 | 494.76 ftii |
| 22 in. | 346.66 ftii | 148.ane ft2 | 494.76 fttwo |
| 24 in. | 349.34 fttwo | 148.one ft2 | 497.44 ft2 |
Skillion Roof
two×four Rafters and two×iv Studs
| Stud spacing | Wall surface area | Roof area | Total expanse |
| xvi inwards. | 373.68 ftii | 147.11 fttwo | 520.79 ft2 |
| xviii in. | 376.64 ftii | 147.xi fttwo | 523.75 ftii |
| twenty inward. | 382.26 ftii | 147.11 ftii | 529.37 ftii |
| 22 inward. | 382.26 fttwo | 147.xi ftii | 529.37 ftii |
| 24 in. | 385.26 ft2 | 147.eleven ft2 | 532.37 ft2 |
2×four Rafters too ii×vi Studs
| Stud spacing | Wall surface area | Roof area | Total area |
| xvi inwards. | 368.34 ft2 | 147.11 ft2 | 515.45 fttwo |
| eighteen in. | 373.98 ftii | 147.xi fttwo | 521.09 ft2 |
| 20 inwards. | 376.94 ft2 | 147.xi ftii | 524.05 fttwo |
| 22 inward. | 376.94 fttwo | 147.11 ft2 | 524.05 fttwo |
| 24 in. | 379.nine fttwo | 147.xi ftii | 527.01 ftii |
two×four Rafters as well as 2×viii Studs
| Stud spacing | Wall expanse | Roof area | Total area |
| 16 inward. | 363.02 ftii | 147.xi ft2 | 510.thirteen ftii |
| xviii inward. | 368.64 fttwo | 147.xi ftii | 515.75 fttwo |
| twenty inwards. | 371.6 fttwo | 147.11 ft2 | 518.71 ft2 |
| 22 inwards. | 371.6 fttwo | 147.eleven ft2 | 518.71 fttwo |
| 24 inwards. | 374.56 ft2 | 147.eleven fttwo | 521.67 ft2 |
two×6 Rafters in addition to two×four Studs
| Stud spacing | Wall surface area | Roof expanse | Total surface area |
| sixteen inwards. | 373.68 fttwo | 143.65 fttwo | 517.33 fttwo |
| eighteen inward. | 376.64 ftii | 143.65 fttwo | 520.29 ftii |
| twenty in. | 382.26 fttwo | 143.65 fttwo | 525.91 ftii |
| 22 inward. | 382.26 ft2 | 143.65 ft2 | 525.91 ftii |
| 24 in. | 385.26 ft2 | 143.65 ft2 | 528.91 fttwo |
ii×half dozen Rafters in addition to 2×vi Studs
| Stud spacing | Wall expanse | Roof surface area | Total area |
| sixteen inward. | 368.34 ft2 | 143.65 fttwo | 511.99 fttwo |
| eighteen in. | 373.98 fttwo | 143.65 ftii | 517.63 ft2 |
| 20 inwards. | 376.94 ft2 | 143.65 ftii | 520.59 ftii |
| 22 inwards. | 376.94 fttwo | 143.65 ft2 | 520.59 ft2 |
| 24 inward. | 379.ix fttwo | 143.65 fttwo | 523.55 ft2 |
2×six Rafters in addition to 2×8 Studs
| Stud spacing | Wall surface area | Roof expanse | Total area |
| 16 inward. | 363.02 ftii | 143.65 ft2 | 506.67 ftii |
| xviii inwards. | 368.64 ft2 | 143.65 fttwo | 512.29 ft2 |
| xx inward. | 371.six fttwo | 143.65 ft2 | 515.25 ftii |
| 22 inward. | 371.6 ft2 | 143.65 fttwo | 515.25 ftii |
| 24 inward. | 374.56 ft2 | 143.65 ftii | 518.21 ft2 |
If yous are insulating a shed, you lot should besides view ventilating it. There are a number of ways to attain this.
Comments
Post a Comment